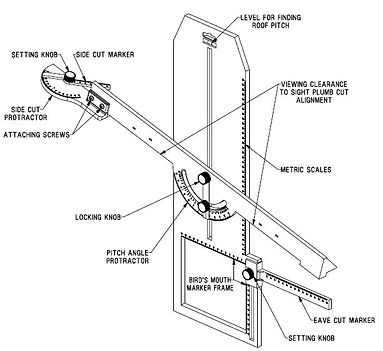
The Roofing Protractor has been invented to solve a problem that roof builders have been struggling with for many years. There are many complex calculations for working out the various angles needed to be cut in the vast array of roof pieces that make up the roof frame so that it will all fit together.

The Roofing Protractor has been designed to make this task quick, simple and most of all accurate so you can save time while ensuring your roof frame will fit together with the high degree of accuracy you require.
 

The Roofing Protractor is ideal for when building the following Roof Types.

Skillion Roof: A Skillion Roof slopes from one side of the building to the other in
a single span and no ridge. As a result the external walls are of varying heights.

Lean-to roof: A roof with one slope only that is built against a higher wall.

Jerkinhead Roof: Consists of a Gable Roof with a truncated Hip Roof
section at the top to reduce the height of the Gable.

Hip and Valley Roof: A type of roof containing sloping planes of the same pitch on each of four sides. A hip roof contains no gables.

Gambrel Roof: A type of roof containing two sloping planes of different pitch on each side of the ridge. The lower plane has a steeper slope than the upper. A gambrel roof usually contains a gable at each end, just like a standard gable roof.

Mansard Roof: A type of roof containing two sloping planes of different pitch on each of four sides. The lower plane has a much steeper pitch than the upper,
often approaching vertical. It contains no Gables.

Gable: The upper portion of a sidewall that comes to a triangular point at the ridge of a sloping roof.

Gable roof: A type of roof containing sloping planes of the same pitch on each side of the ridge. A gable roof typically contains a gable at each end.

Dutch Gable: Is a combination of a Hip roof with a small Gable at the top and a sloping roof below.

Gazebo Roof: Often Hexagonal or Octagonal but usually symmetrical in their design.

​

The underside of the plumb cut can be marked using a chalk line.Jack and Creeper Rafters can also be laid out easily on this bench.For a timber Fascia the tail cut should be done after the roof is built using string lines.Using a metal Fascia the tail cut can be more easily made on the roofbench since the brackets are adjustable.

​

The diagram shows a common rafter spanning half the width of a standard roof. Hence the distance H will equal half the width of the building.The distance X is calculated according to the formula; V = H – T/2.

The H and V value can be used in conjunction withthe tables provided to calculate other lengths.

The Rise R is calculated using the rise height factor according to theformula; R = V x Rise Height Factor.

The lengths L1 and L2 for the positions of the Tail and Bird’s Mouth cuts can be calculated using the common rafter factor according to the formula;L1 = V x Common Rafter Factor.
 

The following is a worked example for a 24° roofpitch and 3 metre half span or run, depending onwhich term you prefer. Dimensions may beworked out in any units whether metric orimperial. For clarity sake the example usesmillimetres since it’s easier if decimal pointswon’t be required.

Some builders prefer to work to the centreline ofthe roof in the middle of the ridge and make anallowance for it’s width at the end, but it is moreaccurate to apply the allowance prior tocalculating the lengths that you need to markyour cuts with the tool.

Hence for a run of 3000 with a 50 thick ridgebeam; V = 3000 – 50/2V = 2975

The Common Rafter Length Factor would betaken from the tables and is 1.095 and the RiseHeight Factor is 0.445

Hence the following calculations

R = 2975 x 0.445

R = 1324.5

L = 2975 x 1.095

L = 3257

By varying the inputs according to the desiredoutcome any of the other dimensions that areshown may also be obtained.

This example shows how Hip or Valley rafters are set out.

Allowances A1 and A2 are usually applied to allow forthe unique geometry of the Hip and Valley.Since they complicate the geometric calculations mostbuilders prefer to measure the length to the Bird’s Mouthin a direct diagonal line after the roof is pitched (built).

For a 24° roof pitch the Hip or Valley angle of 17.48°can be read directly from the tables.

The angle of 47.4° can also be found under the Hip orValley Side Cut Angle column.Mitres in the Hip or Valley seat will also be at this angle.

The Hip or Valley Length Factor can be readily foundin tables under Length Factors.

The lengths L or L1 can be calculated according tothe formulae; L = V x Hip or Valley Length Factor.L1 = (V-A2) x Hip or Valley Length Factor.

If you wish to measure, calculate or approximate theallowances A1 and A2 then by following the diagram youmay calculate the Length L1. This in turn would enable youto cut Hip and Valley rafters directly from the work bench.

Valley seats are almost always mitred as shown, but Hipseats are difficult to cut with the mitre facing inwards so thesimplified version is usually used. To do this the corner ofthe Top plates is usually mitred instead.

​

The length of Creeper or Jack Rafters is reduced accordingto what is known as the common diminishment.

Using the tables provided this length can be calculated asusing the Common Rafter Factor. The example whichfollows is for a 24 degree pitched roof, the common rafterfactor from the tables being 1.095

Therefore; 500mm x 1.095 = 547mm.

Note: All lengths are measured to thepoint of cut.

This picture shows the correct use of theside cut marker set to the common sidecut angle taken from the tables for 24°.

All Purlins are measured for length after the roof is pitched (built).A simple Purlin prop gauge can be used to determine the Proplengths and cutouts.

​

The tool can also be applied simply as a protractor toset out the Purlin Cuts.

The angles are taken directly from the tablesprovided. The diagram shown refers to a 6/12 roofpitch.

Having two lockable protractors is invaluable inproviding the ability to reliably transfer the correctsettings to any number of Purlins.